Number Info for

Crop Image

×

Crop

Count the number of hundreds:

1 hundreds

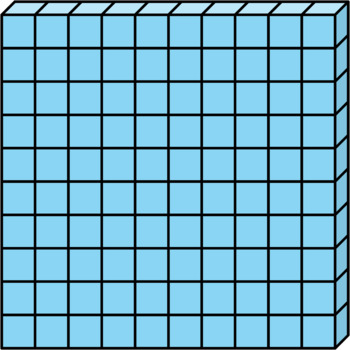
Add up Hundred-Groups

Hundred-Groups = 100

Hundred-Groups = 100

Count the number of tens:

8 tens

Add up Ten-Groups

Ten-Groups = 10 + 10 + 10 + 10 + 10 + 10 + 10 + 10

Ten-Groups = 80

Count the number of ones:

0 ones

Add this to the ones for our total:

180 = 100 Hundreds + 80 Tens + 0 ones

180 = 100 + 80 + 0

Show numerical properties of 180

180

Draw this point on a number line:

Word Notation for 180

one hundred eighty

Write the number 180 in expanded notation form:

Decompose 180

Express in powers of 10

Each digit in the whole number represents a power of 10:

Take the whole number portion on the left side of the decimal

Build Expanded Notation with powers of 10

Expanded Notation of 180 = (1 x 10²) + (8 x 10¹) + (0 x 10⁰)

Expanded Notation of 180 = (1 x 100) + (8 x 10) + (0 x 1)

Prove this is the correct notation:

180 = 100 + 80 + 0

180 = 180 <---- Correct!

Tally Marks for 180

Make blocks of 5

Tally Marks Definition:

1 tally mark = |

2 tally marks = ||

3 tally marks = |||

4 tally marks = ||||

5 tally marks = | | | |

36 blocks of 5 and 0 left over

5 = | | | |

10 = | | | |

15 = | | | |

20 = | | | |

25 = | | | |

30 = | | | |

35 = | | | |

40 = | | | |

45 = | | | |

50 = | | | |

55 = | | | |

60 = | | | |

65 = | | | |

70 = | | | |

75 = | | | |

80 = | | | |

85 = | | | |

90 = | | | |

95 = | | | |

100 = | | | |

105 = | | | |

110 = | | | |

115 = | | | |

120 = | | | |

125 = | | | |

130 = | | | |

135 = | | | |

140 = | | | |

145 = | | | |

150 = | | | |

155 = | | | |

160 = | | | |

165 = | | | |

170 = | | | |

175 = | | | |

180 = | | | |

Tallies for 180

| | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | | |

Ordinal for 180

Define an ordinal number

A position in a list

180th

Digit and Reduced Digit Sum for 180

Calculate the digit sum of 180

Calculate the reduced digit sum of 180

Add up 3 digits of 180:

Digit Sum → 1 + 8 + 0 = 9

Since our digit sum ≤ 9:
we have our reduced digit sum

Digit Sum → 1 + 8 + 0 = 9

Digit Product for 180:

Calculate the digit product of 180

Digit Product = Value when you multiply
all the digits of a number together.

We multiply the 3 digits of 180 together

Digit product of 180 = 1 * 8 * 0

Digit product of 180 = 0

Opposite of 180

Opposite of 180 = -(180)

Opposite of = -180

Place Value for 180

Place value describes each digit

Whole Number Position 3: 180

1 is our hundreds digit

This means we have 1 sets of hundreds

Whole Number Position 2: 180

8 is our tens digit

This means we have 8 sets of tens

Whole Number Position 1: 180

0 is our ones digit

This means we have 0 sets of ones

1 is our hundreds digit
8 is our tens digit
0 is our ones digit

Natural Logarithm of 180

When e^y = x and e = 2.718281828459
We have Ln(x) = log_e(x) = y

Evaluate x = 180

Ln(180) = log_e(180) = 5.1929568508902

Is 180 divisible by:

2,3,4,5,6,7,8,9,10,11

Divisibililty Check for 2

Last digit ends in 0,2,4,6,8

The last digit of 180 is 0

Since 0 is equal to 0,2,4,6,8:
then 180 is divisible by 2

Divisibililty Check for 3

Sum of the digits is divisible by 3

The sum of the digits for 180 is 1 + 8 + 0 = 9

Since 9 is divisible by 3:
Then 180 is divisible by 3

Divisibililty Check for 4

Take the last two digits
Are they divisible by 4?

The last 2 digits of 180 are 80

Since 80 is divisible by 4:
Then 180 is divisible by 4

Divisibililty Check for 5

Number ends with a 0 or 5

The last digit of 180 is 0

Since 0 is equal to 0 or 5:
Then 180 is divisible by 5

Divisibililty Check for 6

Divisible by both 2 and 3

Since 180 is divisible by 2 and 3:
Then 180 is divisible by 6

Divisibililty Check for 7

Multiply each respective digit by 1,3,2,6,4,5
Work backwards
Repeat as necessary

0(1) + 8(3) + 1(2) = 27

Since 27 is not divisible by 7:
Then 180 is not divisible by 7

Divisibililty Check for 8

Take the last three digits
Are they divisible by 8

The last 3 digits of 180 are 180

Since 180 is not divisible by 8:
Then 180 is not divisible by 8

Divisibililty Check for 9

Sum of digits divisible by 9

The sum of the digits for 180 is 1 + 8 + 0 = 9

Since 9 is divisible by 9:
Then 180 is divisible by 9

Divisibililty Check for 10

Ends with a 0

The last digit of 180 is 0

Since 0 is equal to 0:
Then 180 is divisible by 10

Divisibililty Check for 11

Σ odd digits - Σ even digits = 0
or 180 is a multiple of 11

Sum the odd digits:

180

1 + 0

Odd Sum = 1

Sum the even digits:

180

8

Even Sum = 8

Take the difference:

Δ = Odd Sum - Even Sum

Δ = 1 - 8

Δ = -7

Divisibility Check:

Because Δ / 11 = 16.363636363636:
Then 180 is NOT divisible by 11

Divisibility Final Answer

180 is divisible by
(2,3,4,5,6,9,10)

Final Answer